Quantum Pair State Transfer on Isomorphic Branches

Table of Links

Introduction, Acknowledgments and References

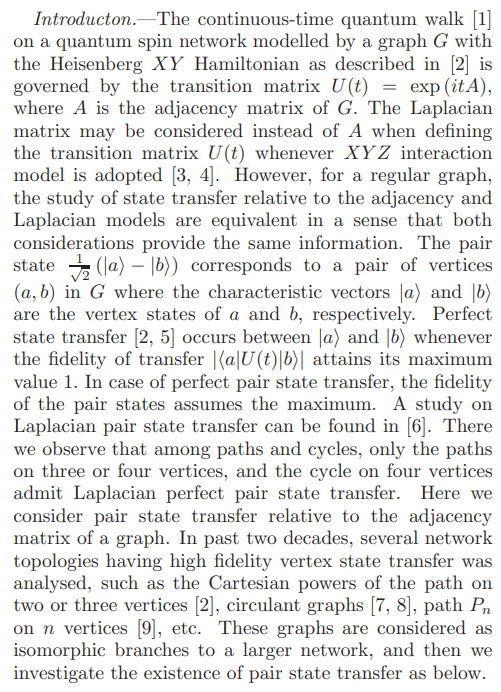
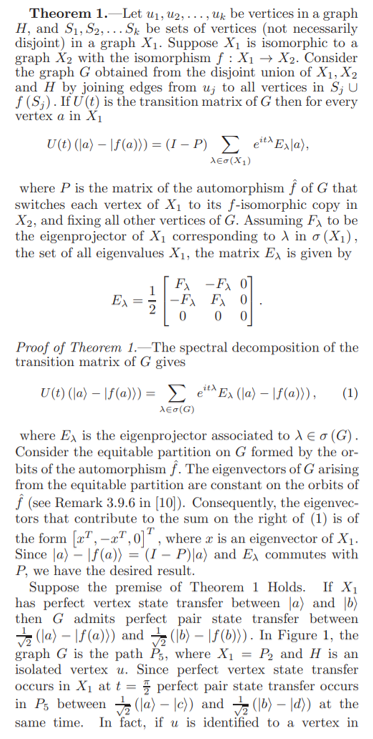
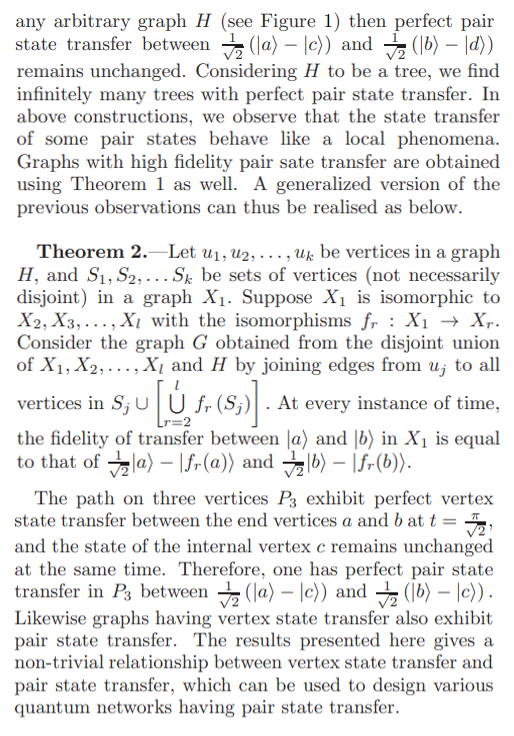
Quantum state transfer plays an important role in quantum information processing. The evolution of certain pair states in a quantum network with Heisenberg XY Hamiltonian depends only on the local structure of the network, and it remains unchanged even if the global structure is altered. All graphs with high-fidelity vertex state transfer may be considered as isomorphic branches of the graph underlying a large quantum network to exhibit high-fidelity pair state transfer. Among other graphs, one may construct infinite family of trees admitting perfect pair state transfer.

ACKNOWLEDGMENTS

The research is funded by Science and Engineering Research Board (Project: SRG/2021/000522).

References

[1] E. Farhi and S. Gutmann, Quantum computation and decision trees, Phys. Rev. A 58, 915 (1998).

[2] M. Christandl, N. Datta, A. Ekert, and A. J. Landahl, Perfect state transfer in quantum spin networks, Phys. Rev. Lett. 92, 187902 (2004).

[3] S. Bose, A. Casaccino, S. Mancini, and S. Severini, Communication in XYZ all-to-all quantum networks with a missing link, Int. J. Quantum Inf. 7, 713 (2009).

[4] H. Pal, Laplacian state transfer on graphs with an edge perturbation between twin vertices, Discrete Math. 345, 112872 (2022).

[5] S. Bose, Quantum communication through an unmodulated spin chain, Phys. Rev. Lett. 91, 207901 (2003).

[6] Q. Chen and C. Godsil, Pair state transfer, Quantum Inf. Process. 19, 321 (2020).

[7] M. Baˇsi´c, Characterization of quantum circulant networks having perfect state transfer, Quantum Inf. Process. 12, 345 (2013).

[8] H. Pal and B. Bhattacharjya, Pretty good state transfer on circulant graphs, Electron. J. Combin. 24, Paper No. 2.23, 13 (2017).

[9] C. Godsil, S. Kirkland, S. Severini, and J. Smith, Number-theoretic nature of communication in quantum spin systems, Phys. Rev. Lett. 109, 050502 (2012).

[10] D. Cvetkovi´c, P. Rowlinson, and S. Simi´c, An introduction to the theory of graph spectra, London Mathematical Society Student Texts, Vol. 75 (Cambridge University Press, Cambridge, 2010) pp. xii+364.